How To Find The Height Of A Flagpole Using Trigonometry

In this topic, we volition exist studying some ways in which trigonometry is used in life effectually you lot.

Trigonometry is one of the near aboriginal subjects studied past scholars all over the world. Trigonometry was invented considering its demand arose in astronomy. Since and so astronomers have used information technology, for case, to calculate distances from the Earth to the planets and stars. Trigonometry is also used in geography and in navigation.

The cognition of trigonometry is used to observe heights of structures, construct maps, make up one's mind the position of an island in relation to the longitudes and latitudes.

What Do You Hateful past Meridian and Distances?

The most pregnant definitions that are used when dealing with heights and distances are given equally:

Line of sight: It is the line drawn from the eye of an observer to the indicate in the object viewed by the observer.

Here, the cat is the observer and the object is the bird.

The angle of elevation: The angle between the horizontal and the line of sight joining an observation betoken to an elevated object.

In the post-obit effigy, the bending of height of the kite from the point \(A\) is \({30^\circ }\), and from point \(B\) is \({60^\circ }\)

The bending of depression: The angle between the horizontal and the line of sight joining an observation point to an object below the horizontal level.

In the post-obit figure, if the top of the building is our observation point, and so the angle of depression of person \(Ten\) is \({45^\circ}\), and that of person \(Y\)  is \({lx^\circ }\).

Allow u.s.a. now feel how trigonometry is applied to practical situations.

Trigonometric ratios can be used to find heights and distances. Some useful relations are illustrated past the below-given diagrams which help us to determine heights and distances.

1. The angles of depression of the meridian and the bottom of an \(8\, yd\) tall building from the superlative of a multi-storeyed building are \(30^\circ \) and \(45^\circ \), respectively. Find the height of the multi-storeyed building and the distance between the two buildings.

   Hint: Endeavor to depict the figure according to a given situation.

How to Notice Pinnacle and Distances?

To measure the heights and distances of different objects, nosotros use trigonometric ratios.

Use the Tangent rule to calculate the height of the tree (in a higher place center level).
tan(bending) = opposite/adjacent
Where the opposite is the acme of the tree and next is the distance between you and the tree.

This is rearranged to:
opposite = tan(angle) ten next

or more simply
\[\text{pinnacle} = \text{tan(bending)} \times \text{distance}\]

Distance tin can exist calculated as:

\(\text{B (distance)} = \dfrac {\text{A (height)}} {\text{tan (e)}}\)

Therefore, to calculate \(B\) (distance) nosotros will demand the value of \(A\) (acme) and angle \(eastward\).

Important Notes

one. The angle between the horizontal and the line of sight joining an observation point to an object beneath the horizontal level is called the bending of elevation.

2. The bending between the horizontal and the line of sight joining an observation point to an object beneath the horizontal level is chosen the bending of depression.

iii. \(\text{B (distance)} = \dfrac {\text{A (height)}} {\text{tan (e)}}\)

Trigonometric Ratios Tabular array

Values of trigonometric functions for the standard angles such as 0°, 30°, 45°, 60°, and 90° could be easily found using a trigonometric ratios table.

The tabular array consists of trigonometric ratios – sine, cosine, tangent, cosecant, secant, and cotangent.

In short, these ratios are written as sin, cos, tan, cosec, sec, and cot.

It is best to think the values of the trigonometric ratios of these standard angles.

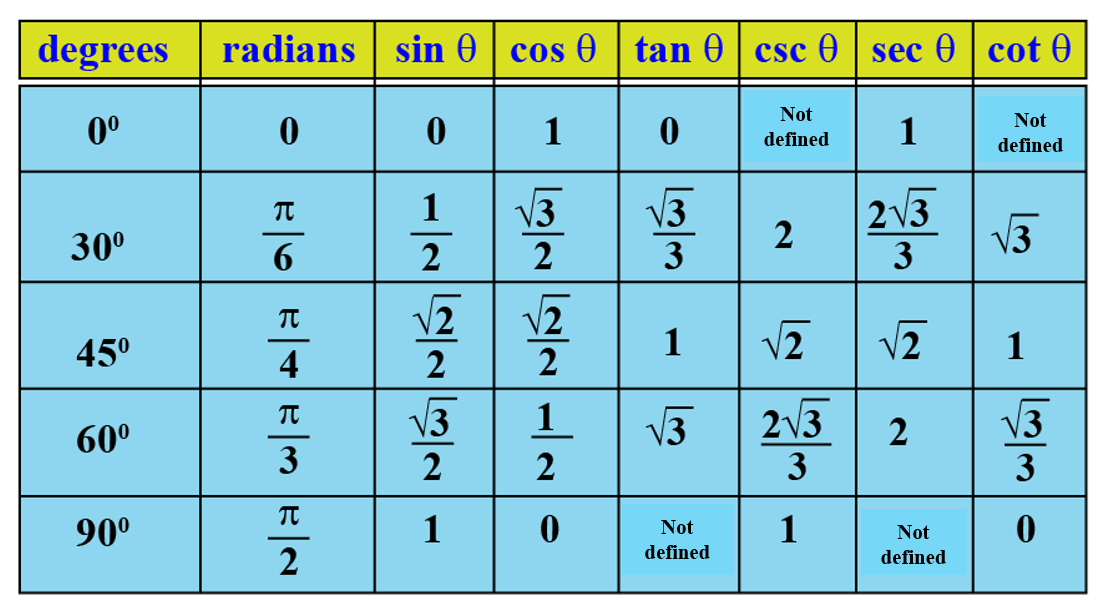
A trigonometric tabular array has wide awarding in fields similar science and engineering.

---

Solved Examples

A homo standing at a certain distance from a building, observe the bending of pinnacle of its top to be \({60^\circ }\). He walks 30 yds away from the edifice. At present, the angle of peak of the building's tiptop is \({30^\circ}\). How high is the building?

Solution

Let the top of the building exist \(h\), and \(d\) be the original distance between the man and the building. The following figure depicts the given situation:

We have:

\[\begin{align}
\tan {60^\circ } &= \sqrt three  \hfill \\
\Rightarrow \frac{h}{d} &= \sqrt 3  \hfill \\
\Rightarrow d &= \frac{h}{{\sqrt 3 }} \hfill \\
\end{marshal} \]

Also,

\[\begin{align}
\tan {30^\circ } &= \frac{1}{{\sqrt 3 }} \hfill \\
\Rightarrow \frac{h}{{d + 30}} &= \frac{1}{{\sqrt three }} \hfill \\
\Rightarrow \sqrt 3 h &= d + 30 \hfill \\
\Rightarrow \sqrt 3 h &= \frac{h}{{\sqrt 3 }} + 30 \hfill \\
\Rightarrow h\left( {\sqrt three  - \frac{1}{{\sqrt three }}} \right) &= thirty \hfill \\
\end{align} \]

\[ \Rightarrow h = 15\sqrt 3 yd \approx 26\;yd\]

 \(\therefore\) The building's height is virtually 26 yards.

From a certain signal on the ground, the angle of meridian of the top of a tree is \(\blastoff \). On moving \(p\)  meters towards the tree, the bending of top becomes \(\beta \) . Bear witness that the height of the tower is

\[h = \left( {\frac{{p\tan \alpha \tan \beta }}{{\tan \beta - \tan \blastoff }}} \right)\;metres\]

Solution

Detect the following effigy, which depicts this situation:

Here, \(d\) and \(h\)  are unknown and we need to detect \(h\) .Nosotros have :

\[\tan \beta = \frac{h}{d} \Rightarrow d = h\cot \beta \]

Now, we accept,

\[\begin{align}
\tan \alpha  &= \frac{h}{{p + d}} \hfill \\
\Rightarrow h &= (p + d)\tan \alpha  \hfill \\
&= (p + h\cot \beta )\tan \alpha  \hfill \\
&= p\tan \blastoff  + h\tan \alpha \cot \beta  \hfill \\
\Rightarrow h\left( {1 - \tan \blastoff \cot \beta } \right) &= p\tan \alpha  \hfill \\
\Rightarrow h &= \frac{{p\tan \alpha }}{{ane - \tan \alpha \cot \beta }} \hfill \\
&= \frac{{p\tan \alpha }}{{1 - \frac{{\tan \alpha }}{{\tan \beta }}}} \hfill \\
\end{align} \]

\[ h = \dfrac{p\tan \blastoff \tan \beta }{\tan \beta  - \tan \blastoff }\]

 \(\therefore\) \( h = \dfrac{p\tan \alpha \tan \beta }{\tan \beta - \tan \blastoff }\)

A house has a window \(h\) yards above the footing. Beyond the street from this house, in that location is a alpine pole. The angle of elevation and depression of the top and bottom of this pole from the window are \(\theta \) and \(\varphi \) respectively. Make up one's mind the height of the pole.

Solution

The following effigy depicts the given situation:

Notation that \(d = h\cot \varphi \) and

\[{h_1} = d\tan \theta = h\tan \theta \cot \varphi \]

Thus, the height of the pole is,

\[\brainstorm{gathered}
H = h + {h_1} \hfill \\
\Rightarrow H = h(1 + \tan \theta \cot \varphi ) \hfill \\
\end{gathered} \]

\(\therefore\) The height of the pole is \(= h(1 + \tan \theta \cot \varphi ) \)

From an observation tower, the angle of depression of two cars on the opposite side of the belfry are \(\blastoff \)and \(\beta \). If the tower's height is \(h\) yards, detect the distance between the cars.

Solution

Notice the post-obit figure:

Nosotros have,

\[d_1 = h\cot \alpha, \quad d_2 =h\cot \beta\]

Thus, the distance between the cars is,

\[D = {d_1} + {d_2} = h\left( {\cot \alpha + \cot \beta } \right)\,yd\]

\(\therefore\) The distance between the cars is\(= h\left( {\cot \alpha + \cot \beta } \right)\,yd\)

---

I nteractive Questions

Hither are a few activities for you to practice. Select/Type your answer and click the "Check Answer" button to see the issue.

---

Let's Summarize

This mini-lesson targeted the fascinating concept of heights and distances. The math journey around the heights and distances starts with what a educatee already knows, and goes on to creatively crafting a fresh concept in the immature minds. Done in a way that not only information technology is relatable and piece of cake to grasp, but as well will stay with them forever. Here lies the magic with Cuemath.

About Cuemath

At Cuemath, our team of math experts is defended to making learning fun for our favorite readers, the students!

Through an interactive and engaging learning-education-learning approach, the teachers explore all angles of a topic.

Be it problems, online classes, incertitude sessions, or whatever other form of relation, it's the logical thinking and smart learning approach that nosotros, at Cuemath, believe in.

---

Frequently Asked Questions

ane. How practice yous find the distance in trigonometry?

\(\text{B (distance)} = \dfrac {\text{A (height)}} {\text{tan (e)}}\)

Therefore, to summate \(B\) (distance) we will need the value of \(A\) (height) and angle \(e\).

ii. What is an bending of low in trigonometry?

If a person stands and looks down at an object, the angle of depression is the angle between the horizontal line of sight and the object.

3. What is the formula for the angle of depression?

If a person stands and looks down at an object, the bending of depression is the angle between the horizontal line of sight and the object.

4. Is the angle of elevation equal to low?

An angle of acme of one location relative to another is always coinciding (equal in mensurate) to the bending of low of the first location relative to the second.

v. What is the bending of sight in trigonometry?

The angle of meridian of an object as seen by an observer is the angle between the horizontal and the line from the object to the observer'southward eye (the line of sight).

vi. What is the human relationship betwixt height and altitude?

Using trigonometry, if we are provided with any of the two quantities that may be a side or an angle, nosotros can calculate all the rest of the quantities. By the police of alternate angles, the angle of peak and angle of depression are consequently equal in magnitude (α = β). Tan α is equal to the ratio of the top and distance.

7. What type of triangle is used to calculate heights and distances?

A right-angled triangle is used to calculate heights and distances.

eight. What is the angle of summit case?

Source: https://www.cuemath.com/trigonometry/heights-and-distances/

Share this post